Academics
Careers
What Is Computational Fluency?
Computational fluency is described as the ability to compute with accuracy, flexibility, and efficiency (Adding It Up, 2001). Computational fluency for every individual is developed over time. Fluency is not a fixed ability. Rather, it grows and develops through ample opportunities to practice and discuss strategies. We demonstrate fluency when we estimate solutions, use mental mathematics, use pencil and paper, and even when we decide to use calculators. The strategies we use are often dictated by the numbers we need to compute.
The way that we think about numbers and computation is as unique as our fingerprints. Even so, there are strategies for computation that many of us share.
How is it developed?
Computational fluency is developed over time. It begins before we enter school and is developed throughout a lifetime. In elementary grades, we learn about the four operations, the properties of the operations, and strategies used to perform the operations. We apply this understanding to make computation more efficient. We learn that the numbers we are trying to calculate help us determine what strategy would be most efficient.
It is developed through concrete models (blocks), drawings, and then symbols (numbers). Teachers model strategies and tools students can use to acquire understanding. Teachers facilitate discussion to exchange ideas and perspectives when working with computation.
Important Notes for Parents and Teachers
Computational fluency is not fixed. Students can develop and improve their fluency through exposure, experience, and discussion.
Basic fact fluency supports computational fluency but frequently the two are co-developed.
Students are exposed to different strategies to develop flexibility and efficiency.
Students are not required to solve a problem with a particular strategy or multiple strategies.
Students should have freedom to choose the most efficient strategy for them.
Students should be able to explain how they found their solution. Explanations should not be required for every calculation. But these explanations are useful as fluency is developed so misunderstandings can be corrected.
Algorithms can be an efficient method of computation. This is especially true when numbers are large or unfriendly. Use of paper/pencil and/or algorithms is connected to the numbers being calculated and the strategies of the individual. For example, 199 + 82 may be more efficiently solved mentally by taking 1 from 82 to make a new problem 200 + 81. Other examples such as 14,325 + 9,180 may be better solved with an algorithm.
Why do we need to do things differently?

What is an Empty Number Line?
The empty number line, or open number line as it is sometimes referred to, was originally proposed as a model for addition and subtraction by researchers from the Netherlands in the 1980s. A number line with no numbers or markers, essentially the empty number line is a visual representation for recording and sharing students’ thinking strategies during the process of mental computation.
Before using an empty number line students need to show a secure understanding of numbers to 100. Prior experiences counting on and back using number lines, recall of addition and subtraction facts for all numbers to ten and the ability to add/subtract a multiple of ten to or from any two-digit number are all important prerequisite skills.
Introducing the Empty Number Line
During this stage students practice moving to given numbers in the least number of jumps. Possible questions may include:
How can we go from 0 to 59 in the least number of jumps of tens and ones?
How can we go from 0 to 189 in the least number of jumps of hundreds, tens and ones?
Students should be encouraged to share different strategies and discuss which strategy is the most efficient. For example, when jumping from 0 to 59 one student could make five jumps of ten and nine jumps of one, while another may make 6 jumps of ten to 60 and then jump back one to 59.
Using the Empty Number Line to Solve Addition and Subtraction Problems
During the next phase students solve addition and subtraction problems and draw the jumps to explain their thinking process. Students are free to choose what type of jumps they will use. Again the focus is on sharing different strategies in order to lead students to use the empty number line efficiently when adding or subtracting any pairs of numbers. Possible questions include:
How can we go from 27 to 53 in a small number of jumps? Who has another way?
How can we go from 62 to 45 in a small number of jumps? Who has another way?
How can we solve 34+23? (counting on without crossing the tens boundary)
How can we solve 37 + 25? (counting on crossing the tens boundary)
How can we solve 47 - 23? (counting back without crossing the tens boundary)
How can we solve 42-25? (counting back crossing the tens boundary)
How can we solve 82- 47? (counting up)
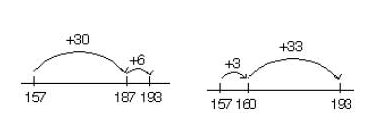
How can we solve 157 + 36?
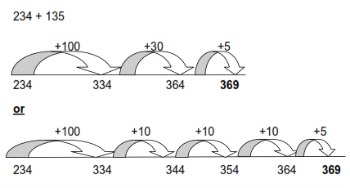
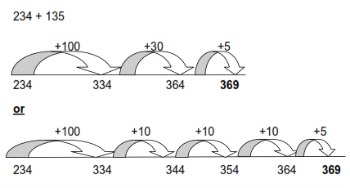
One of the interesting things about mental calculations is that we do not all think the same way. The empty number line allows students to see the variety of ways that the same question can be solved. For example, to solve 157 + 36 one student may begin at 157, add 30, then 6 while another may start at 157 and break the 36 into 3 and 33. This turns the question into the problem of adding 33 to 160. Writing equations horizontally forces students to look at the numerals, whereas written vertically students tend to immediately turn to the procedural algorithm.
Using the Empty Number Line to Solve Word Problems
Once students are confident with using the number line for showing their thinking strategy they can use it to support them while solving a range of problems in different contexts (e.g. elapsed time, money, measurement).
Addition (2 digit + 2 digit)
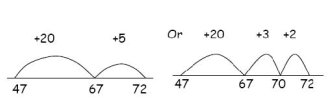
A sunflower is 47 cm tall. It grows another 25cm. How tall is it?
Subtraction (2 digit – 2 digit)
I need 72 dollars to buy a skateboard. I have 39 dollars already. How many more dollars do I need to save?
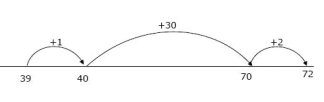
39 is placed near the start of the empty number line and 72 near the end. We can count up in 'friendly' jumps to reach 72. First a jump of 1 to reach 40 (multiples of ten are easy numbers to jump to and from), then a jump of 30 to reach 70 and finally a jump of 2 to reach our target of 72. I need to save 33 more dollars.
Subtraction (2 digit - 2 digit)
A piece of string is 42cm long. If you cut off 25cm how much will be left?
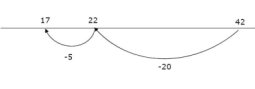
42 is placed towards the end of the empty number line. 20 is subtracted to get to 22, then 5 is subtracted to get to 17.
Subtraction (3 digit – 3 digit)
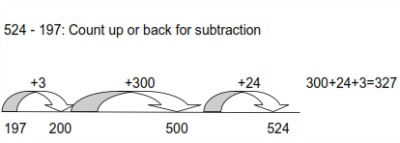
There are 543 people on the subway platform. 387 board a train. How many people are left on the platform?
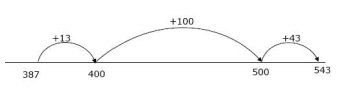
We start at 387 and count up in 'friendly' jumps to reach 543. First a jump of 13 to reach 400 (multiples of ten and hundred are easy numbers to jump to and from) then a jump of 100 to reach 500 and finally a jump of 43 to reach the target of 543. 156 people are left on the platform.
Multiplication:
Example: Use an empty number line to calculate 19 x 4
20 (a ‘friendly’ number) is one more than 19. We can do 4 jumps of 20 to 80 and then jump back 4 spaces to 76. 19 x 4 = 76
How could you use this idea of multiplying by 20 and adjusting to solve these problems?: 21 x 6; 19 x 8; 21 x 4
Can you use the idea of adjusting by one to calculate multiples of 29 and 31?
Division (2 digit by 1 digit)
6 cupcakes fit in a box. How many boxes can be filled with 85 cupcakes?
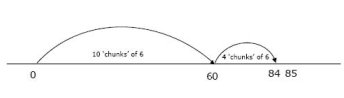
We need to find out many sixes there are in 85. To solve this problem we could count up in sixes one group at a time but this would not be efficient. We need to think about easy, but bigger ‘chunks’ of 6 such as 6x10(60). We can add 6 chunks of 6 as 60 and then add a further 4 chunks of 6 (24) to total 84, leaving 1 spare cupcake. 14 boxes can be filled.
Division (3 digit by 2 digit)
If a box holds 28 apples, how many boxes can be filled with 350 apples?
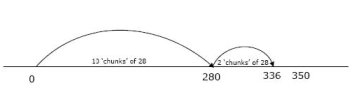
To solve this problem we need to find out how many 28s there are there in 350. We can think about ‘friendly’ chunks of 28 such as 28 x 10 (280). First the 10 chunks of 28 (280) are added then two more ‘chunks’ of 28 (56) are added mentally to leave 14 chocolates. This is not enough to fill a further box so 12 boxes can be filled.
Given ongoing opportunities to use the empty number line students will begin to solve problems mentally by picturing the empty number line in their heads. Regular use increases students’ confidence in their ability to use numbers flexibly and leads more easily towards mental calculations without paper.
Place Value Strategies
Place value strategies are used by students prior to working with the standard algorithm. As students work with place value strategies they develop a conceptual understanding of number, place value, and properties. The understanding and fluent use of place value strategies work to develop a strong sense of number which is critical for higher level mathematics.
So, what do place value strategies look like? Many different strategies based on place value exist for both written and mental computations. Some examples of written methods for Grades 1-5 are shown below.Prior to developing fluency with standard algorithms the CCSS emphasize place value strategies. This strong focus on place value strategies plays a critical role in the development of mental and written computation strategies, while providing students with the opportunity to develop a deep understanding of how the standard algorithms work. Common Core Standards explicitly referencing strategies based on place value include 1.NBT4, 2.NBT.5, 2.NBT.6, 2.NBT.7, 3.NBT.2, 3.NBT.3, 4.NBT.5, 4.NBT.6, 5.NBT.6, and 5.NBT.7.
Possible 1st Grade Place Value Strategies:
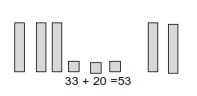
Draw Base 10 blocks: 33 + 20 = 53
45 + 30 =75
Draw jumps on an empty number line: 2 digit + multiple of 10
Possible 2nd Grade Place Value Strategies:
769
Draw jumps on an empty number line:
Partial Sums (Expanded form layout): Each addend is represented using expanded notation. Like place values are added or subtracted.
123 + 234 = 238 + 473 =
100 + 20 + 3 200 + 30 + 8
+ 200 + 30 + 4 + 400 + 70 + 3
300 + 50 + 7 = 357 600 + 100 + 11= 711
548 - 325 614 - 459
500 + 40 + 8 600 + 10 + 4 becomes 500 + 100 + 14
- 300 + 20 + 5 - 400 + 50 + 9 - 400 + 50 + 9
200 + 20 + 3 = 223 100 + 50 + 5= 155
Possible 3rd Grade Place Value Strategies:
Partial Sums: Expanded Form layout as above
or vertical format.
632+325=
632
+ 325
900
50
7
957
Partial Differences: Each number is represented using expanded notation. Like place values aregrouped and subtracted. Negative place values may result.
752 - 436 523-259=
700 + 50 +2 500 + 20+ 3
- 400+30+6 - 200+ 50+9
300+ 20 - 4 = 316 300 - 30 - 6 = 264
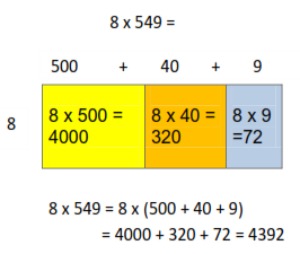
Use multiplication facts and place value
to multiply by multiples of ten: 9 x 80 =
9 x80 = 9 x 8 tens
= 72 tens = 720
9 x80 = 720
Use the distributive property to multiply within 100: 15 x 5 =
15 x 5 = (10 x 5) + (5x5)
= 50 + 25
= 75
Possible 4th Grade Place Value Strategies:
Partial Products: (2 digit x 2 digit)
32
x 34
900 (30 x 30)
120 (30 x 4)
60 (2 x 30)
8 (2 x 4)
1,088
Area Model: (1 digit x 3 digit)
769
769
Area Model: (2 digit x 2 digit)
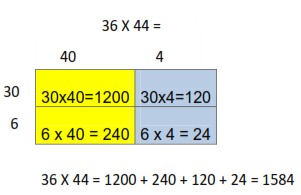
769
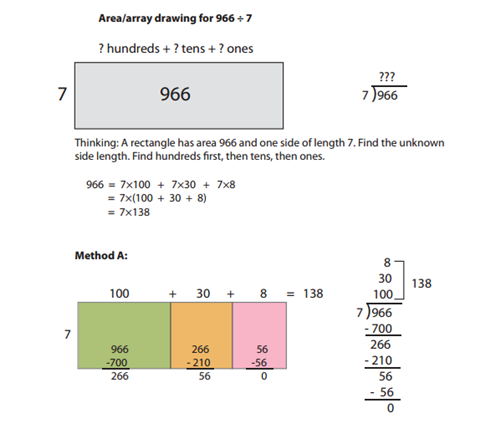
Partial Quotients: 7725/6
1204 r 1
6) 7225
- 6000 ( 1000 x 6)
1225
- 1200 (200 x 6)
25
- 24 (4 x 6)
1
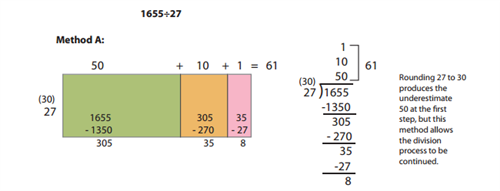
Partition the Dividend: Partition the dividend into multiples of the divisor.
292/4
70 + 3 = 73
4) 280 + 12
Possible 5th Grade Place Value Strategies:
Add decimals on an empty number line:
35.8 + 8.3 =
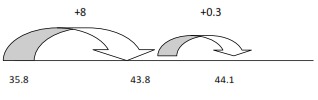
Subtract decimals on an empty number line: (Count up to find the difference)
126.4 - 58.7 =
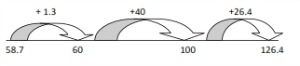
Start at 58.7 and jump up 1.3 to 60, then jump 40 to 100, then jump 26.4 to 126.4. Add the jumps:
40 + 26.4 + 1.3 = 67.7
Draw Base-Ten Blocks: Division with decimals

769
Area Model: Multiplying decimals
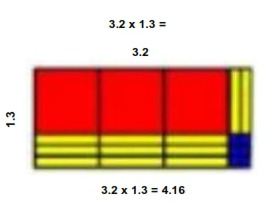
769
Examples of strategies used in Grade 1 Click Here
Examples of strategies used in Grade 2 Click Here
Examples of strategies used in Grade 3 Click Here
Examples of strategies used in Grade 4 Click Here
Examples of strategies used in Grade 5 Click Here